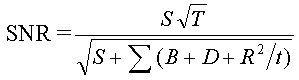
An imaging sensor is essentially a photon counting device. Signal to noise ratio (SNR) can be calculated by summing over all the pixels within an aperture as follows:
where:
S = signal in photons
B = sky background in photons
D = dark current in electrons
R = readout noise in electrons
T = total integration time in seconds
t = integration time per image in seconds
A signal-to-noise ratio of three is usually considered the minimum for detection. A higher SNR is required for photometric measurements.
This has several implications for astrophotography:
1. Best SNR performance is achieved by keeping the star size small, subject to the Nyquist criterion (at least 2.5 pixels across FWHM).
2. On very short exposures, or sequences of short exposures, the readout noise performance may be the limiting factor.
3. In most cases, for long exposures the sky background is the limiting factor for performance, even at dark sky sites.
4. If properly calibrated, dark current noise for cooled cameras is usually only important for narrowband imaging (spectroscopy, narrowband interference filters, etc.). This is especially true if you eliminate hot pixels through dither and sigma clip.
To improve performance in the read-noise limited case, consider increasing the exposure, particularly if summing a sequence of exposures.
In all cases, increasing telescope size, camera quantum efficiency, or exposure time will improve your limiting magnitude (faintest detectable objects).